GRE Math Flashcards
Perfect Squares 1-15
1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144, 169, 196, 225
How to recognize if a # is a multiple of 12
The sum of the digits it a multiple of 3 and the last two digits is a multiple of 4. (i.e 144: 1+4+4=9 which is a multiple of 3, and 44 is a multiple of 4, so 144 is a multiple of 12.)
How to recognize a # as a multiple of 9
The sum of the digits is a multiple of 9.
Slope given 2 points
m= (Y1-Y2)/(X1-X2)
How to recognize a multiple of 6
Sum of digits is a multiple of 3 and the last digit is even.
How to recognize a # as a multiple of 4
The last 2 digits are a multiple of 4. (i.e 144 …. 44 is a multiple of 4, so 144 must also be a multiple of 4.)
How to recognize a # as a multiple of 3
The sum of the digits is a multiple of 3
When dividing exponential #s with the same base, you do this to the exponents…
Subtract them.
When multiplying exponential #s with the same base, you do this to the exponents…
Add them.
First 10 prime #s
2, 3, 5, 7, 11, 13, 17, 19, 23, 29
Find distance when given time and rate
d=rt so r= d/t and t=d/r
binomial product of (x+y)(x-y)
x²-y²
factored binomial product of (x+y)²
x²+2xy+y²
factored binomial product of (x-y)²
x²-2xy+y²
binomial product of (x+y)²
(x+y)(x+y)
binomial product of (x-y)²
(x+y)(x-y)
3 What is the relationship between lengths of the sides of a triangle and the measure of the angles of the triangle?
Sides with the same lengths are opposite angles with the same measure.
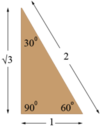
1 What is an important property of a 30-60-90 triangle?
The triangle is a right triangle.
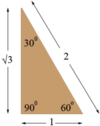
2 What is an important property of a 30-60-90 triangle?
The hypotenuse is twice the length of the shorter leg.
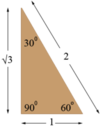
3 What is an important property of a 30-60-90 triangle?
The ratio of the length of the three sides is x:x√3:2x
The negative exponent x⁻ⁿ is equivalent to what?
1/xⁿ
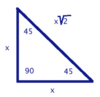
1 What are the important properties of a 45-45-90 triangle?
The triangle is a right triangle.
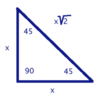
2 What are the important properties of a 45-45-90 triangle?
The triangle is isosceles (AC=BC).

3 What are the important properties of a 45-45-90 triangle?
The ratio of the lengths of the three sides is x:x:x√2.
formula for distance problems
distance=rate×time or d=rt
The sum of the angles in a quadrilateral is
360°
The sum of the measures of the n angles in a polygon with n sides
(n-2) x 180

In any polygon, all external angles equal up to
360°

In a Regular Polygon, the measure of each exterior angle
360/n

The consecutive angles in a parallelogram equal
180°

A quadrilateral where two diagonals bisect each other
Parallelogram

In a rectangle, all angles are
Right
Area of a Parallelogram:
A=(base)(height)

(x-y)(x+y)
x²-y²
(x-y)²
x²-2xy+y²
(x+y)²
x²+2xy+y²
An Angle that’s 180°
Straight Angle
The sum of all angles around a point
360°
If a pair of parallel lines is cut by a transversal that’s not perpendicular, the sum of any acute angle and any obtuse angle is
180
Distance
(rate)(time) d=rt
If a lamp increases from $80 to $100, what is the percent increase?
= 25%.
= (actual increase/original amount) x 100%
= 20/80 x 100% = 1/4 x 100% = 25%
The percent decrease of a quantity
= (actual decrease/Original amount) x 100%
If a lamp decreases to $80, from $100, what is the decrease in price?
= (actual decrease/Original amount) x100%
= 20/100x100% = 20%
To increase a number by x%
multiply by 1+x%
To decrease a number by x%
multiply by 1-x%
If a>b then
-a<-b
Probability of an Event
P(E) = number of favorable outcomes/total number of possible outcomes
If Event is impossible
P(E) = ø
Probability of Event all cases
∅≤P(E)≤1
Probability of E not occurring:
1 - P(E)
Circumference of a circle
pi(diameter)
Circumference of a circle
2(pi)r
Area of a circle
(pi)r²
Volume of a rectangular solid
(length)(width)(height)
Vertical lines
Do not have slopes!
Any Horizontal line slope
zero
X is the opposite of
-X
The only number that is equal to its opposite
∅ ∅=∅
7 divided by ∅
Null
∅ Is neither
Positive or Negative
Consecutive integers
x, x+1, x+2
One is (a prime or not?)
NOT A PRIME
Positive integers that have exactly 2 positive divisors are
Prime numbers (2, 3, 5, 7, 11, 13, 17, 19, 23)
∅ Is
EVEN
∅ is a multiple of
zero is a multiple of every number, BUT zero is NOT a FACTOR of any number except zero
∅ is a multiple of
Every number
2 is the only
Even prime number
bⁿ
b∧b∧b (where b is used as a factor n times)
2⁵*2³
2⁸
2⁵/2³
2²
(2²)³
2⁶
2³×7³
(2x7)³
∅²
∅
If a is positive, aⁿ is
Positive
If a is negative and n is even then aⁿ is (positive or negative?)
aⁿ is positive
-3²
9
-3³
-27
If a
a+c
1ⁿ
1
1 is a divisor of
every number
1 is the
smallest positive integer
25^(1/2) or sqrt. 25 =
5 OR -5
What are the real numbers?
All the numbers on the number line (negative, rational, irrational, decimal, integer). All the numbers on the GRE are real. (-2, 1, .25, 1/2, pi)
What are the rational numbers?
All numbers which can be expressed as a ratio of two integers. (All integers and fractions.) (-2, 1, .25, 1/2)
What are the irrational numbers?
All real numbers which can’t be expressed as a ratio of two integers, positive and negative (pi, -sqrt3)
What are the integers?
All numbers multiples of 1.
10
11, 13, 17, 19
20
23, 29
30
31, 37
40
41, 43, 47
50
53, 59 note 57=3x19
60
61, 67
70
71, 73, 79
1/8 in percent?
12.50%
1/6 in percent?
16.67%
3/8 in percent?
37.50%
5/8 in percent?
62.50%
7/8 in percent?
87.50%
5/6 in percent?
83.33%
x^6 / x^3
x^(6-3) = x^3
0^0
undefined
Can you add sqrt 3 and sqrt 5?
No, only like radicals can be added.
Can you subtract 3sqrt4 from sqrt4?
Yes, like radicals can be added/subtracted.
(6sqrt3) x (2sqrt5) =
(6 x 2)(sqrt3 x sqrt5) = 12sqrt15
(12sqrt15) / (2sqrt5) =
(12/2) x (sqrt15 / sqrt5) = 6sqrt3
Can you simplify sqrt72?
Yes, because you can factor out a perfect square (36). Sqrt(36 x 2) = sqrt36 X sqrt2 = 6sqrt2.
10^6 has how many zeroes?
6
To multiply a number by 10^x
move the decimal point to the right x places
Define a “term”,
A term is a numerical constant or the product (or quotient) of a numerical constant and one or more variables. (3x, 4x^2 and 2a/c)
Define an “expression”.
An algebraic expression is a combination of one of more terms. Terms in an expression are separated by either addition or subtraction signs. (3xy, 4ab, -5cd, x^2 + x - 1)
Define a “monomial”
An expression with just one term (-6x, 2a^2)
Solve the quadratic equation ax^2 + bx + c= 0
x = [(-b)+/- (sqrt b^2 - 4ac)]/2a
What is the “domain” of a function?
The set of input values for a function.
What is the “range” of a function?
The set of output values for a function.
What is the “range” of a series of numbers?
The greatest value minus the smallest.
How to find the circumference of a circle which circumscribes a square?
Circumference = Diameter(pi). Use pythagorean theorem to find the diagonal of the square (the diameter).
When does a function automatically have a restricted domain (2)?
When we need to avoid having a zero in the denominator or avoid taking the square root of a number.
The larger the absolute value of the slope…
the steeper the slope.
Which quadrant is the upper right hand?
I
Which quandrant is the lower right hand?
IV
Which quadrant is the upper left hand?
II
Which quadrant is the lower left hand?
III
What are “supplementary angles?”
Two angles whose sum is 180.
What is a chord of a circle?
A chord is a line segment joining two points on a circle.
What is a central angle?
A central angle is an angle formed by 2 radii.
What is a tangent?
A tangent is a line that only touches one point on the circumference of a circle.
What is an arc of a circle?
An arc is a portion of a circumference of a circle.
What is a minor arc?
The shortest arc between points A and B on a circle’s diameter.
What is a major arc?
The longest arc between points A and B on a circle’s diameter.
Formula to calculate arc length?
Arc length = (n/360) x pi(2r) where n is the number of degrees.
Formula for the area of a sector of a circle?
Sector area = (n/360) X (pi)r^2
What is the “solution” for a system of linear equations?
The point of intersection of the systems.
What is the “solution” for a set of inequalities.
The overlapping sections.
What is it called when a point is reflected to the quadrant opposite it (i.e. I to III or II to IV)?
A reflection about the origin.
What is the graph of f(x) shifted upward c units or spaces?
f(x) + c
What is the graph of f(x) shifted downward c units or spaces?
f(x) - c
What is the graph of f(x) shifted left c units or spaces?
f(x + c)
What is the graph of f(x) shifted right c units or spaces?
f(x-c)
What are complementary angles?
Two angles whose sum is 90.
What are congruent triangles?
Triangles with same measure and same side lengths.
Legs: 3, 4. Hypotenuse?
5
Legs 6, 8. Hypotenuse?
10
Legs 5, 12. Hypotenuse?
13
Evaluate and write as a mixed number: 2/7 - 3/21 + 2 & 4/14
2 & 3/7
8.84 / 5.2
1.7
Evaluate 4/11 + 11/12
1 & 37/132
200
3: 200, 240, 280
What number between 70 & 75, inclusive, has the greatest number of factors?
72
What are the smallest three prime numbers greater than 65?
67, 71, 73
Which is greater? 64^5 or 16^8
16^8
Evaluate (4^3)^2
4096
Write 10,843 X 10^7 in scientific notation
1.0843 X 10^11
True or false? 4.809 X 10^7 = .0004809 X 10^11
TRUE
If a=-1 and b=3, what is the value of (4(a^3)(b^2) - 12(a^2)(b^5)) / (16(a^3)(b^2))?
20.5
T or F? Given d,e &f =/ 0, [(d^3)e(f^5)] / 2d(e^3) / [3(d^2)(e^3)(f^7)] / [6(e^5)(f^2)]?
TRUE
Simplify 4sqrt21 X 5sqrt2 / 10sqrt7
(4x5/10)xsq(21x2/7)=2sqrt6
Simplify 9^(1/2) X 4^3 X 2^(-6)?
3
Reduce: 4.8 : 0.8 : 1.6
6:01:02
Ratio of ages of Anna and Emma is 3:5 and of Emma and Nicolas is 3:5. What is the ratio of Anna to Nicholas’ ages?
9:25
What percent of 40 is 22?
55%
Convert 0.7% to a fraction.
7 / 1000
Hector invested $6000. Part was invested in account with 9% simple annual interest, and the rest in account with 7% simple annual interest. If he earned $490 in the first year of these investments, how much did he invest in each account?
$3,500 in the 9% and $2,500 in the 7%.
Employee X is paid 19.50 per hour no matter how many a week. Employee Y earns 18 for the first 40 and 1.5 the hourly wage for every hour after that. If both earned the same amount and worked the same in one week, how many did each work?
48
The perimeter of a square is 48 inches. The length of its diagonal is:
12sqrt2
If Madagascar’s exports totaled 1.3 billion in 2009, and 4% came from China, what was the value in millions of the country’s exports to China?
52
Whats the difference between factors and multiples?
Factors are few, multiples are many.
How many multiples does a given number have?
Infinite.
P and r are factors of 100. What is greater, pr or 100?
Indeterminable.
If r, t, s & u are distinct, consecutive prime numbers, less than 31, which of the following could be an average of them (4, 4.25, 6, 9, 24, 22, 24)
4.25, 6, 22
Is 0 even or odd?
Even
How many digits are there between the decimal point and the first even digit in the decimal equivalent of 1/[(2^8)(5^3)]
0
What are the roots of the quadrinomial x^2 + 2x + 1?
The two xes after factoring.
Factor x^2 - xy + x.
x(x - y + 1)
Simplify the expression [(b^2 - c^2) / (b - c)]
(b + c)
Simplify (a^2 + b)^2 - (a^2 - b)^2
4a^2(b)
What is the common monomial factor in the expression 4(c^3)d - (c^2)(d^2) + 2cd?
cd
Simplify the expression (p^2 - q^2)/ -5(q - p)
(p + q)/5
What is the coefficient of the x^2 term in the product of (x + 1)(x + 2)(x -1)?
2
(a^-1)/a^5
1/a^6
x^2 = 9. What is the value of x?
3, -3
6w^2 - w - 15 = 0
-3/2 , 5/3
5x^2 - 35x -55 = 0
[(7+ sqrt93) /2], [(7 - sqrt93) / 2]
If 10800 is invested at a simple interest rate of 4%, what is the value of the investment after 18 months?
$11,448
If 4500 is invested at a simple interest rate of 6%, what is the value of the investment after 10 months?
4725
What is the maximum value for the function g(x) = (-2x^2) -1?
-1
For what values should the domain be restricted for the function f(x) = sqrt(x + 8)
-8
What transformation occurs if point C is reflected over the x-axis and then the y-axis?
A reflection about the axis.
The four angles around a point measure y, 2y, 35 and 55 respectively. What is the value of y?
90
For similar triangles, the ratio of their corresponding sides is 2:3. What is the ratio of their areas?
4:9. The ratio of the areas of two similar triangles equals the square of the ratio of the corresponding sides.
What is the ratio of the sides of an isosceles right triangle?
1:1:sqrt2
What is the ratio of the sides of a 30-60-90 triangle?
1:sqrt3:2
1:sqrt3:2 is the ratio of the sides of what kind of triangle?
A 30-60-90 triangle.
What is the side length of an equilateral triangle with altitude 6?
4sqrt3. The triangle can be divided into two equal 30-60-90 triangles with side 6 as the side in which 6 = xsqrt3. So x =2sqrt3…
In a triangle where the two legs are 4 and 3, what is the value of a line directly intersecting the middle coming from the meeting point of the two legs?
2.4. We calculate the area (6) and then turn the triangle on its side and use x as the height to calculate again. (5x)/2=6
Describe the relationship between 3x^2 and 3(x - 1)^2
The graph of 3(x - 1)^2 is a translation (shift) of the graph one unit or space to the right.
Describe the relationship between the graphs of x^2 and (1/2)x^2
The second graph is less steep.
Suppose that the graph of f(x) is the result of stretching y=x + 5 away from the x-axis by a factor of 2. What is the new equation for the graph f(x)?
y = (x + 5)/2
Suppose that the graph of f(x) is the result of sliding the graph of y=2x^2 down 3 units of spaces. What is the new equation?
y = 2x^2 - 3
What is an exterior angle?
An angle which is supplementary to an interior angle.
What is the measure of an exterior angle of a regular pentagon?
72
The ratio of the areas of two similar polygons is …
… the square of the ratios of the corresponding sides.
In similar hexagons, the ratio of the areas is 16:25. What is the ratio of their corresponding sides?
4:05
What is the area of a regular hexagon with side 6?
54sqrt3. (divide the hexagon into 6 congruent equilateral triangles.
A triangle is inscribed in a semi circle with legs 5 and 12. What is the circumfermence of the semicircle?
13pi / 2
A cylinder has a surface area of 22pi. If the cylinder has a height of 10, what is the radius?
1
What is the third quartile of the following data set: 44, 58, 63, 63, 68, 70, 82
70
If the 80th percentile of the measurements is 72degrees, about how many measurments are between 69 degrees and 72 degrees? Round your answer to the nearest tenth
18
The objects in a set are called two names:
members or elements
What are the members or elements of a set?
The objects within a set.
What is a finite set?
A set with a number of elements which can be counted.
What is the name of set with a number of elements which cannot be counted?
An infinite set.
What is a subset?
a grouping of the members within a set based on a shared characteristic.
What is the name for a grouping of the members within a set based on a shared characteristic?
A subset.
What is the empty set?
A set with no members, denoted by a circle with a diagonal through it.
What is a set with no members called?
the empty set, denoted by a circle with a diagonal through it.
What is the “union” of A and B?
The set of elements which can be found in either A or B.
What is the intersection of A and B?
The set of elements found in both A and B.
If you have a set of n objects, but you only want to order k of them, what formula do you use to determine the number of permutations?
n! / (n-k)!
Suppose you have a set of n objects, and you want to select k of them, but the order doesn’t matter. What formula do you use to determine the number of combinations of n objects taken k at a time?
n! / (k!)(n-k)!
How many 3-digit positive integers are even and do not contain the digit 4?
288 (8 * 9 * 4)
From a box of 12 candles, you are to remove 5. How many different sets of 5 candles could you remove?
12! / 5!7! = 792
There are 10 finalists for the school spelling bee. A first, second, and third place trophy will be awarded. In how many ways can the judges award the 3 prizes?
10! / (10-3)! = 720
There are 10 finalists for the school spelling bee. A first, second, and third place trophy will be awarded. How many different people can get the three prizes?
10! / 3!(10-3)! = 120
A company places a 6-symbol code on each product. The code consists of the letter T, followed by 3 numerical digits, and then 2 consonants (Y is a conson). How many codes are possible?
441000 = 1 * 10 * 10 * 10 * 21 * 21
Nine coins are tossed simultaneously. In how many of the outcomes will the fourth coin tossed show heads?
2^9 / 2 = 256
Find the surface area of a cylinder with radius 3 and height 12.
90pi
What is the surface area of a cylinder with radius 5 and height 8?
130pi
A cylinder has surface area 22pi. If the cylinder has a height of 10, what is its radius?
1
What is the ratio of the surface area of a cube with an edge of 10 to the surface area of a rectangular solid with dimensions 2, 4, and 6?
75:11:00
A brick with dimensions 10. 15 and 25 weighs 1.5 kg. A second brick (same density) has dimensions 12, 18, and 30. What is the weight of the second brick?
2.592 kg
If 8 schools are in a conference, how many games are played if each team plays each other exactly once?
- n = 8, k = 2. n! / k!(n-k)!
Which is greater? 27^(-4) or 9^(-8)
27^(-4)
Which is greater? 200x^295 or 10x^294?
Relationship cannot be determined (what if x is negative?)


