Formulas, etc Flashcards
1
Q
A
2
Q
A
3
Q
A
4
Q
A
5
Q
A
6
Q
A
7
Q
A
8
Q
A
9
Q
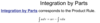
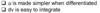
Integration by Parts
A
10
Q
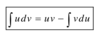
Integration by Parts - Steps
A
11
Q
A
12
Q
A
13
Q
A
14
Q
A
15
Q
A
16
Q
A
17
Q
A
18
Q
A
19
Q
A
20
Q
A
21
Q
A
22
Q
A
23
Q
A
24
Q
A
25
26
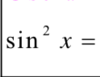
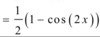
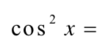
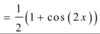
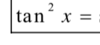
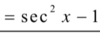
To perform a PFD
Degree of the Numerator \< Degree of the Denominator.
If it is not, one must perform long division first.
Q(x) must be factored completely:
only linear and/or irreducible quadratic factors.
27
Case I: Q(x) is the product of distinct linear factors.
28
Case II: Q(x) is the product of linear factors where some are repeated.
29
Case III: Q(x) contains irreducible quadratic factors that are not repeated.
30
Case IV: Q(x) contains a repeated irreducible quadratic factors.
31
Determine the coefficients - Method 1: Substitute in (1) values of x. Start with the roots of Q(x)
32
Determine the coefficients:
Method 2: Expand the right hand side of (1), collect like terms and match the coefficients of the powers of x.
