Equity and Fixed Income (value debt sec/yield spot fwd/interest rate risk/credit anal)

Question 1

Explain steps in the bond valuation process

Answer 1

To value a bond, one must:

• Estimate the amount and timing of the bond’s future payments of interest and principal
• Determine the appropriate discount rate(s)
• Calculate the sum of the PVs of the bond’s cash flows

Question 2

Describe types of bonds for which estimating cash flows is difficult.

Answer 2

Certain bond features, including embedded options, convertibility, or floating rates, can make the estimation of future cash flows uncertain, which adds complexity to the estimation of bond values.

Question 3

Calculate the value of a bond (coupon and zero-coupon).

Answer 3

To compute the value of an option-free coupon bond, value the coupon payments as an annuity and add the preset value of the principal repayment at maturity.

The value of a zero-coupon bond calculated using a semiannual discount rate, i (one-half its annual yield to maturity), is:

bond value = maturity value / (1 + i)^{number of years * 2}

Question 4

Compute the value of a 10-year, $1,000 face value zero-coupon bond with a yield to maturity of 8%

Answer 4

To find the value of this bond given its yield to maturity of 8% (a 4% semiannual rate), we can calculate:

bond value = 1,000 / (1 + 0.08/2)1^0*2 = 1,000 / (1.04)²⁰ = $456.39

Or use the calc

The difference between the current price of the bond ($456.39) and its par value ($1,000) is the amount of compound interest that will be earned over the 10-year life of the issue.

Question 5

Explain how the price of a bond changes if the discount rate changes and as the bond approaches its maturity date

Answer 5

The interest rates (yields) do not change, a bond’s price will move toward its par value as time passes and the maturity date approaches.

To compute the change in value that is attributable to the passage of time, revalue the bond with a smaller number of periods to maturity.

Question 6

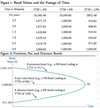
Calculate the change in value of a bond given a change in its discount rate.

Answer 6

The change in value that is attributable to a change in the discount rate can be calculated as the change in the bond’s PV based on the new discount rate (yield)

Question 7

A bond has a par value of $1,000, a 6% semiannual coupon, and three years to maturity. Compute the bond values when the yield to maturity is 3%, 6%, and 12%.

Answer 7

At I/Y = 3/2; N = 3*2; FV = 1,000; PMT = 60/2; CPT - PV = -1,085.458

At I/Y = 6/2; N = 3*2; FV = 1,000; PMT = 60/2; CPT - PV = -1,000.000

At I/Y = 12/2; N = 3*2; FV = 1,000; PMT = 60/2; CPT - PV = -852.480

If the yield to maturity equals the couon rate, the bond value is equal to par. If the yield to maturity is higher (lower) than the coupon rate, the bond is trading at a discount (premium) to par.

We can now calclate the percentage change in price for changes in yield. If the required yield decreases from 6% to 3%, the value of the bond increases by:

1,085.46/1,000.00 - 1 = 8.546%

If the yield increases from 6% to 12%, the bond value decreases by:

852.48/1,000.00 - 1 = -14.752%

Question 8

Explain and demonstrate the use of the arbitrage free valuation approach and describe how a dealer can generate an arbitrage profit if a bond is mispriced

Answer 8

A Treasury spot yield curve is considered “arbitrage-free”, if the PV of Treasury securities calculated using these rates are equal to equilibrium market prices.

if bond prices are not equal to their arbitrate-free values, dealers can generate arbitrage profits by buying the lower-priced alternative (either the bond or the individual cash flows) and selling the higher-priced alternative (either the individual cash flows or a package of the individual cash flows equivalent to the bond).

Question 9

Consider a 6% Treasury note with 1.5 years to maturity. Spot rates (expressed as yield to maturity) are: 6 months = 5%, 1 year = 6%, and 1.5 years = 7%. If the note is selling for $992, compute the arbitrage profit, and explain how a dealer would perform the arbitrage.

Answer 9

To value the note, note that the cash flows (per $1,000 par value) will be $30, $30, and $1030, and the semiannual discount rates are half the stated yield to maturity.

Using the semiannual spot rates, the PV of the expected cash flows is:

PV using spot rates = 30/1.025 + 30/1.03² + 1,030/1.035³ = $986.55

This value is less than the market price of the note, so we will buy the individual cash flows (zero-coupon bonds), combine them into a 1.5 year note package, and sell the package for the market price of the note. This will result in an immediate and riskless profit of 992.00 - 986.55 = $5.45 per bond

Question 10

Describe the sources of return from investing in a bond

Answer 10

Debt securities that make explicit interest payments have three sources of return:

1. The periodic coupon interest payments made by the issuer
2. The recovery of principal, along with any capital gain or loss that orruces when the bond matures, is called, or is sold
3. Reinvestment income, or the income earned from reinvesting the periodic coupon payments (i.e., the compound interest on reinvested coupon payments).

Question 11

Calculate and interpret traditional yield measures for fixed-rate bonds and explain their limitations and assumptions.

Answer 11

Yield to maturity (YTM) for a semiannual-pay coupon bond is calculated as two times the semiannual discount rate that makes the PV of the bond’s promised cash flows equal to its market price plus accrued interest. For an annual-pay coupon bond, the YTM is simply the annual discount rate that makes the PV of the bond’s promised cash flows equal to its market price plys accrued interest.

The current yield for a bond is its annual interest payment divided by its market price.

Yield to call (put) is calculated as the YTM but with the number of periods until the call (put) and the call (put) price substituted for the number of periods to maturity and the maturity value.

The cash flow yield is a monthly internal rate of return based on a presumed prepayment rate and the current market price of a mortgage-backed or asset-back security.

These yield measures are limited by their common assumptions that: (1)all cash flows can be discounted at the same rate; (2) the bond will be held to maturity, with all coupons reinvested to maturity at a rate that equals the bond’s YTM; and (3) all coupons payments will be made as scheduled.

Question 12

Consider a 20-year, $1,000 par value, 6% semiannual-pay bond that is curretnly trading at $802.07. Calculate the current yield

Answer 12

The annual cash coupon payment total:

annual cash coupon payment = par value * stated coupon rate = $1,000 * 0.06 = $60

Because the bond is trading at $802.07, the current yield is:

current yield = 60/802.07 = 0.0748, or 7.48%

Note that the current yield is based on annual coupon intrest so that it is the same for semiannual-pay and annual pay bond with the same coupon rate and price.

Question 13

Consider a 20-year, $1,000 par value bond, with 6% coupon rate (semiannual payments) with a full price of $802.07. Calculate the YTM.

Answer 13

Use calc.

PV = -802.07; N = 20*2; FV = 1,000; PMT = 60/2 = 30; CPT I/Y = 4.00

4% is the semiannual discount rate, YTM/2 in the formula, so the YTM = 2*4% = 8%

YTM/2 or semiannual YTM is the same as bond equivalent yield

Question 14

Consider an annual-pay 20-year, $1,000 par value, with a 6% coupon rate and a full price of $802.07. Calculate the annual-pay YTM.

Answer 14

The relationship between the price and the annual-pay YTM on this bond is:

PV = -802.07; N=20; FV = 1,000; PMT = 60; CPT I/Y = 8.019;

8.019% is the annual-pay YTM

Question 15

A 5-year Treasury STRIP is priced at $768. Calculate the semiannual-pay YTM.

Answer 15

PV = -768; FV = 1,000; PMT = 0; N = 10 CPT I/Y = 2.675% * 2 = 5.35% for the semiannual-pay YTM, and PV = -768; FV = 1,000; PMT = 0; N = 5; CPT I/Y = 5.42% for the annual-pay YTM.

The annual-pay YTM of 5.42% means the $768 earning compound interest of 5.42% per year would grow to $1,000 in five years.

Question 16

Consider a 20-year, 10% semiannual-pay bond with a full price of 112 that can be called in five years at 102 and called at par in seven years. Calculate the YTM, YTC, and yield to first par call.

Note: Bond prices are often expressed as a percent of par (e.g., 100 = par)

Answer 16

The YTM can be calculated as: N = 40; PV = -112; PMT = 5; FV = 100; CPT I/Y = 4.361% * 2 = 8.72% = YTM

To compoute the yield to first call (YTFC), we substitute the number of semiannual periods until the first call date (10) for N, and the first call price (102) for FV, as follows:

N = 10; PV = -112; PMT = 5; FV = 102;

CPT I/Y = 3.71% and 2 * 3.71 = 7.42% = YTFC

To calculate the yield to first par call (YTFPC), we will substitute the number of semiannual periods until the first par call date (14) for N and par (100) for FV as follows:

N=14; PV=-112; PMT = 5; FV = 100

CPT I/Y = 3.873% * 2 = 7.746% = YTFPC

Note that the yield to call, 7.42%, is significantly lower than the yield to maturity, 8.72%. If the bond were trading at a discount to par value, there would be no reason to calculate the yield to call. For a discount bond, the YTC will be higher than the YTM since the bond will appreciate more rapidly with the call to at least par and, perhaps, an even greater call price. Bond yield are quoted on a yield to call basis when the YTC is less than the YTM, which can only be the case for bonds trading at a premium to the call price.

Question 17

Consider a 3-year, 6%, $1,000 semiannual-pay bond. The bond is selling for a full price of $925.40. The first put opportunity is at par in two years. Calculate the YTM and the YTP.

Answer 17

Yield to maturity is calculated as:

N = 6; FV = 1,000; PMT = 30; PV = -925.40; CPT I/Y = 4.44 * 2 = 8.88% = YTM

Yield to put is calculated as:

N = 4; FV = 1,000; PMT = 30; PV = -925.40; CPT I/Y = 5.11 * 2 = 10.22% = YTP

Question 18

Explain the reinvestment assumption implicit in calculating yield to maturity and describe the factors that affect reinvestment risk.

Answer 18

YTM is not the realized yield on an investment unless the *reinvestment rate is equal to the YTM. *

The amount of reinvestment income required to generate the YTM over a bond’s life is the difference between the purchase price of the bond, compounded at the YTM until maturity, and the sum of the bond’s interest and principal cash flows.

Reinvestment risk is higher when the coupon rate is greater (maturity held constant) and when the bond has longer maturity (coupon rate held constant)

Question 19

If you purchase a 6%, 10-year Treasury bond at par, how much reinvestment income must be generated over its life to provide the investor with a compound return of 6% on a semiannual basis?

Answer 19

Assuming the bond has a par value of $100, we first calculate the total value that must be generated ten years 920 semiannual periods) from now as:

100(1.03)²⁰ = $180.61

There are 20 bond coupons of $3 each, totaling $60, and a payment of $100 of principal at maturity.

Therefore, the required reinvestment income over the life of the bond is:

180.61 - 100 - 60 = $20.61

Question 20

Calculate and interpret the bond equivalent yield of an annual-pay bond and the annual-pay yield of a semiannual-pay bond

Answer 20

The bond equivalent yield of an annual-pay bond is:

BEY = [√(1 + annual-pay YTM) - 1] * 2

The annual-pay yield can be calculated from the YTM of a semiannual-pay bond as:

EAY = (1 + semiannual-pay YTM/2)² - 1

Question 21

Suppose that a corporation has a semiannual coupon bond trading in the US with a YTM of 6.25%, and an annual coupon bond trading in Europe with a YTM of 6.30%. Which bond has the greater yield?

Answer 21

To determine the answer, we can convert the yield on the annual-pay bond to a (semi-annual) bond equivalent yield. That is:

BEY of an annual-pay bond = [(1 + annual YTM)^1/2 - 1] * 2

Thus, the BEY of the 6.30% annual-pay bond is:

[(1+0.0630)^0.5 - 1] * 2 = [1.031 - 1] * 2 = 0.031 * 2 = 0.062 = 6.2%

The 6.25% semiannual-pay bond provides the better (bond equivalent) yield.

Alternatively, we could convert the YTM of the semiannual-pay bond (which is a bond equivalent yeild) to an equivalent annual-pay basis. The equivalent annual yield (EAY - sometimes known as the effective annual yield) to the 6.25% semi annual-pay YTM is:

equivalent annual yield = (1 + 0.0625/2)²- 1 = 0.0635 is 6.35%

Question 22

Describe the calculation of the theoretical Treasury spot rate curve and calculate the value of a bond using spot rates

Answer 22

The theoretical Treasury spot rate curve is derived by calculating th espot rate for each successive period N based on the spot rate for period N - 1 and the market price of a bond with N coupon payments.

To compute the value of a bond using sport rates, discount each separate cash flow using the spot rate corresponding to the number of periods until the cash flow is to be received.

You are responsible for “describeing” this calculation, not for computing theoretical spot rates

Question 23

Given the following spot rates (in BEY form):

0. 5 years = 4%
1. 0 years = 5%
2. 5 years = 6%

Calculate the value of a 1.5-year, 8% Treasury bond.

Answer 23

Simply lay out the cash flows and discount by the spot rates, which are one-half the quoted rates since they are quoted in BEY form.

4/(1 + 0.04/2)¹ + 4/(1 + 0.05/2)² + 104/(1 + 0.06/2)³ = 102.9

or with TVM function

N=1; PMT=0; I/Y=2; FV=4; CPT PV = -3.92

N=2; PMT=0; I/Y=2.5; FV=4; CPT PV = -3.81

N=3; PMT=0; I/Y=3; FV=104; CPT PV = -95.17

Add values together to get 102.9

Question 24

Explain nominal, zero-volatility, and option-adjusted spreads and the relations among these spreads and option cost.

Answer 24

Three commonly used yield spread measures:

• Nominal spread: bond YTM - Treasury YTM
• Zero-volatility spread (Z-spread or static spread): the equal amount of additional yield that must be added o each Treasury spot rate to get spot rates that will produce a present value for a bond equal to its market price
• Option adjusted-spread (OAS): spread to the spot yield curve after adjusteing for the effects of embedded options. OAS reflects the spread for credit risk and liquidity risk primarily

There is no difference between the nominal and Z-spread when the yield curve is flat. The steeper the spot ield curve and the earlier bond principal is paid (amortizing securities), the greater the difference in the two spread measures.

The option cost for a bond with an embedded option is Z-spread - OAS

For callable bonds, Z-spread > OAS and option cost > 0

For putable bonds, Z-spread < OAS and option cost < 0

Question 25

1-, 2-, and 3-year spot rates on Treasuries are 4%, 8.167%, and 12.377%, respectively. Consider a 3-year, 9% annual coupon corporate bond trading at 89.464. The YTM is 13.50%, and the YTM of a 3-year Treasury is 12%. Compute the nominal spread and the zero-volatility spread of the corporate bond.

Answer 25

The nominal spread is:

nominal spread = YTMBond - YTMTreasury = 13.50 - 12.00 = 1.50%

To compute the Z-spread, set the present value of the bond’s cash flows equal to today’s market price. Discount each cash flow at the appropriate zero-coupon bond spot rate plus a fixed spread equal ZS. Solve for ZS in the following equation and you have the Z-spread:

89.464 = 9/(1.04 + ZS)¹ + 9/(1.08167 + ZS)² + 109/1.12377 + ZS)³

ZS = 1.67% or 167 basis points

Note that this spread is found by trial-and-error. In other words, pick a number “ZS”, plug it into the right-hand side of the equation, and see if the result equals 89.464. If the right-hand side equals the left, then you have found the Z-spread. If not, pick another “ZS” and start over.

This is not a calculation you are expected to make; this example is to help you understand how a Z-spread differs from a nominal spread

Question 26

Suppose you learn that a bond is callable and has an OAS of 135bp. You also know that similar bonds have a Z-spread of 167 basis points. Compute the cost of the embedded option.

Answer 26

The option cost = Z-spread - OAS = 167 - 135 = 32 basis points

Question 27

Explain a foward rate and calculate spot rates from forward rates, forward rates from spot rates, and the value of a bond using forward rates.

Answer 27

Forward rates are current lending/borrowing rates for short-term loans to be made in future periods.

A spot rate for a maturity of N periods is the geometic mean of forward rates over the N periods. The same relation can be used to solve for a forward rate given spot rates for two different periods.

To value a bond using forward rates, dicount the cash flows at times 1 through N by the product of one plus each forward rate for periods 1 to N, and sum them.

S₃ = [(1 + ₁f₀)(1 + ₁f₁)(1 + ₁f₂)]^1/3 - 1

borrowing for three years at the 3-year rate or borrowing for 1-year periods, three years in succession, should have the same cost.

₁f₁ is the rate for a 1-year loan one year from now

₁f₂ is the rate for a 1-year loan to be made two years from now

Question 28

If the current 1-year rate is 2%, the 1-year forward rate (₁f₁) is 3% and the 2-year forward rate (₁f₂) is 4% what is the 3-year spot rate?

Answer 28

S₃ = [(1.02)(1.03)(1.04)]^1/3 - 1 = 2.997%

This can be interpreted to mean that a dollar compounded at 2.997% for three years would produce the same ending value as a dollar that earns compound interest of 2% the first year, 3% the next year, and 4% for the third year.

Question 29

The 2-period spot rate, S₂, is 8% and the current 1-period (spot) rate is 4% (this is both S₁ and ₁f₀). Calculate the forward rate for one period, one period from now, ₁f₁.

Answer 29

(1 + S₂)² = (1 + S₁)(1 + ₁f₁) = (1 + S₂)²/(1 + S₁) - 1 = ₁f₁

or, becuase we know that both choices have the same payoff in two years:

(1.08)² = (1.04)(1 + ₁f₁)

(1 + ₁f₁) = (1.08)²/(1.04)

₁f₁ = (1.08)²/(1.04) - 1 = 1.1664/1.04 = 12.154%

In other words, investors are willing to accept 4.0% on the 1-year bond today (when they could get 8.0% on the 2-year bond today) only because they can get 12.154% on a 1-year bond one year from today. This future rate that can be locked in today is a foward rate.

Similarily, we can back other forward rates out of the spot rates. We know that:

(1 + S₃)³ = (1 + S₁)(1 + ₁f₁)(1 + ₁f₂)

And that:

(1 + S₂)² = (1 + S₁)(1 + ₁f₁), so we can write (1 + S₃)³ = (1 + S₂)²(1 + ₁f₂)

This last equation says that investing for three years at the 3-year spot rate hsould produce the same ending value as investing for two years at the 2-year spot rate and then for a third year at ₁f₂, the 1-year forward rate, two years from now.

Solving for the forward rate, ₁f₂ we get:

(1 + S₃)³/(1 + S₂)² - 1 = ₁f₂

Question 30

The current 1-year rate (1f0) is 4%, the 1-year forward rate for lending from time = 1 to time = 2 is 1f1 = 5%, and the 1 -year forward rate for lending form time = 2 to time = 3 is 1f2 = 6%. Value a 3-year annual-pay bond with a 5% coupon and a par value of $1,000

Answer 30

bond value = 50/(1 + ₁f₀) + 50/((1+₁f₀)(1+₁f₁)) + 1,050/((1+₁f₀)(1+₁f₁)(1+₁f₂))

50/1.04 + 50(1.04)(1.05) + 1,050/(1.04)(1.05)(1.06) = $1,000.98

Question 31

Distinguish between the full valuation approach (the scenario analysis approach) and the duration/convexity approach for measuring interest rate risk, and explain the advantage of using the full valuation approach.

Answer 31

The full valuation approach to measuring interest rate risk involves using a pricing model to value individual bonds and can be used to find th eprice impact of any scenario of interest rate/yield curve changes. It’s advantages are its flexibility and precision.

The duration/convexity approach is based on summary measures of interest rate risk and, while simpler to use for a portfolio of bonds than the full valuation approach, is theoretically correct only for parallel shifts in the yield curve.

Question 32

Consider two option-free bonds. Bond X is an 8% annual-pay bond with five years to maturity, priced at 108.4247 to yield 6% (N=5; PMT=8.00; FV=100; I/Y=6.00%; CPT PV = -108.4247

Bond Y is 5% annual-pay bond with 15 years to maturity, priced at 81.7842 to yield 7%.

Assume a $10 million face-value position in each bond and two scenarios. The first scenario is a parallel shift in the yield curve of +50 basis points. The second scenario is a parallel shift of +100 basis points. Note that the bond price of 108.4247 is the price per $100 of par value. With $10 million of par value bonds, the market value will be $10.84247 million

Use the full valuation approach

Answer 32

The full valuation approach for the two simple scenarios is illustarated in the figure.

Portfolio value change 50bp: (18.41657 - 19.02089) / 19.02089 = -0.03177 = -3.18%

Portfolio value change 100bp: (17.84218 - 19.02089) / 19.02089 = -0.06197 = -6.20%

It’s worth noting that, on an individual bond basis, the effect of an increase in yield on the bond’s values is less for Bond X than for Bond Y (i.e., with a 50bp increase in yields, the value of Bond X falls by 2.02%, whiule the value of Bond Y falls by 4.71%; and with a 100bp increase, X falls by 3.99%, while Y drops by 9.12%). This, of course, is totally predictable since Bond Y is a longer-term bond and has a lower coupon - both of which mean more interest rate risk.

note:

• higher coupon means lower duration (price sensitivity)
• longer maturity means higher duration
• higher market yield means lower duration

Question 33

Describe the price volatility characteristics for option-free, callable, prepayable, and putable bonds when interest rates change.

Answer 33

Callable bonds and prepayable securities will have less price volatility (lower duration) at low yields, compared to option-free bonds.

Putable bonds will have less price volatility at high yields, compated to option-free bonds.

Question 34

Describe positive convexity and negative convexity, and their relation to bond price and yield.

Answer 34

Option-free bonds have a price-yield relationship that is curved (convex toward the origin) and are said to exhibit positive convexity. In this case, bond prices fall less in response to an increase in yield than they rise in response to an equal-sized decrease in yield.

Callable bonds exhibit negative convexity at low yield levels. In this case, bond prices rise less in response to a decrease in yield than they fall in response to an equal sized increase in yield.

Question 35

Calculate and interpret the effective duration of a bond, given information about how the bond’s price will increase and decrease for given changes in interest rates.

Answer 35

Formula for calculating the effective duration of a bond is:

effective duration = (bond price when yields fall - bond price when yields rise) / 2(initial price)*(change in yield in decimal form)

Question 36

Consider a 20-year, semiannual-pay bond with an 8% coupon that is curretnly priced at $908.00 to yield 9%. If the yield declines by 50 basis points (to 8.5%), the price will increase to $952.30, and if the yield increases by 50 basis points (to 9.5%), the price will decline to $866.80. Based on these price and yield changes, calculate the effective duration of this bond.

Answer 36

Begin by computing the average of the percentage change in the bond’s price for the yield increase and the percentage change in price for a yield decrease.

average % price change = ($952.30 - $866.80) / 2*$908.00) = 0.0471 or 4.71%

The 2 in the denominator is to obtain the average price change, and the $908 in the denominator is to obtain this average change as a percentage of the current price.

Our estimate of duration is:

0.0471/0.005 = 4.71%/0.50% = 9.42 = duration

So effective duration is

= ($952.3 - $866.8) / 2*$908*0.005 = 9.416

The interpretation of this result is that 1% change in yield produces an approximate change in the price of this bond of 9.42%.

KNOW THIS FORMULA

To help consider the following:

The price increase in response to a 0.5% decrease in rates was $44.30/$908 = 4.879%

The price decrease in response to a 0.5% increase in rates was $41.20/$908 = 4.537%

The average of the percentage price increase and the percentage price decrease is 4.71%. Becuase we used a 0.5% change in yield to get the price changes, we need ot double this and get a 9.42% change in price for a 1% change in yield. The duration is 9.42.

Question 37

Calculate the approximate percentage price change for a bond, given the bond’s effective duration and a specified change in yield.

Answer 37

percentage change in bond price = -effective duration * change in yield in percent

Question 38

What is the expected percentage price change for a bond with an effective duration of nine in response to an increase in yield of 30 basis points?

Answer 38

-9 * 0.3% = -2.7%

We expect the bond’s price to decrease by 2.7% in response to the yield change. If the bond were priced at $980, the new price is

980 * (1 - 0.027) = $953.54

Question 39

Distinguish among the alternative defintions of duration and explain why effective duration is th emost appropriate measure of interest rate risk for bonds with embedded options.

Answer 39

The most intuitive interpretation of duration is the percentage change in a bond’s price for a 1% change in yield to maturity.

Macaulay duration and modified duration are based on a bond’s promised cash flows.

Effective duration is appropriate for estimating price changes in bonds with embedded options on a bond’s cash flows.

Question 40

Calculate the duration of a portfolio, given the duration of the bonds comprising the portfolio, and explain the limitations of portfolio duration.

Answer 40

The duration of a bond portfolio is equal to a weighted average of the individual bond durations, where the weights are the proportions of total portfolio value in each bond position.

Portfolio duration is limited because it gives the sensitivity of portfolio value only to yield changes that are equal for all bonds in the portfolio, an unlikely scenario for most portfolios.

portfolio duration = w₁D₁ + w₂D₂ + …+w_ND_N

Question 41

Suppose you have a two-security portfolio containing Bonds A and B. The market value of Bond A is $6,000, and the market vluae of Bond B is $4,000. The duration of Bond A is 8.5, and the duration of Bond B is 4.0. Calculate the duration of the portfolio.

Answer 41

First, find the weights of each bond. Becuase th emarket vluae of the portfolio is $10,000 = $6,000 + $4,000, the weight of each security is as follows:

weight in Bond A = $6,000 / $10,000 = 60%

weight in Bond B = $4,000 / $10,000 = 40%

Using the formula for the duration of a portfolio, we get:

portfolio duration = (0.6 * 8.5) + (0.4*4.0) = 6.7

Question 42

Describe the convexity measure of a bond and estimate a bond’s percentage price change, given the bond’s duration and convexity and a specified change in interest rates.

Answer 42

Because of convexity, the duration measure is a poor approximation of price sensitivity for yield changes that are not absolutely small. The convexity adjustment accounts for the curvature of the price-yield relationship.

Incorporating both duration and convexity, we can estimate the percentage change in price in response to a change in yield of (Δy)

={[-duration * (Δy)] + [convexity * (Δy)²]} * 100

with Δy as a decimal and “*100” to get a percent

Question 43

Consider an 8% Treasury bond with a current price of $908 and a YTM of 9%. Calculate the percentage change in price of both a 1% increase and a 1% decrease in YTM based on a duration of 9.42 and a convexity of 68.33.

Answer 43

The duration effect = 9.42 * 0.01 = 0.0942 = 9.42%

The convexity effect = 68.33 * 0.01² * 100 = 0.00683 * 100 = 0.683%

The total effect from a decrease in yield of 1% (from 9% to 8%) is 9.42% + 0.683% = +10.103%

and the estimate of the new price of the bond is 1.10103 * 908 = 999.74. This is much closer to the actual price of the $1,000 than our estimate with only duration

The total effect from an increase in yield of 1% (from 9% to 10%) is -9.42% + 0.683% = -8.737%, and estimate of the bond price is (1-0.08737)(908) = $828.67. Again, this is much closer to the actual price ($828.40) than the estimate based soley on duration.

Question 44

Distinguish betweeen modified convexity and effective convexity

Answer 44

Effective convexity considers expected changes in cash flows that may occur for bonds with embedded optoins, while modified convexity does not

(like effective and modified duration)

Question 45

Calculate the price value of a basis point (PVBP), and explain its relationship to duration.

Answer 45

Price value of a basis point (PVBP) is an estimate of the chcange in a bond’s or a bond portfolio’s value for a one basis point change in yield.

price value of a basis point = duration * 0.0001 * bond value

Question 46

A bond has a market value of $100,000 and a duration of 9.42. What is the price value of a basis point?

Answer 46

Using the duration formula, the percentage change in the bond’s price for a change in yield of 0.01% is 0.01% * 9.42 = 0.0942%. We can calculate 0.0942% of hte original $100,000 portfolio vluae as 0.000942 * 100,000 = $94.20. If the bond’s yield increases (decreases) by on basis point, the portfolio value will fall (rise) by $94.20. $94.20 is the (duration-based) price value of a basis point for this bond.

We could also directly calcualte the price value of basis point for this by increasing the YTM by 0.01% (0.0001) and calculating the change in bond value, This would give us the PVBP for an increase in yield. This would be very close to our duration-based estimate because duration is very good estimate of interest rate risk for small changes in yield. We can ignore the convexity adjustment here because it is of very small magnitude:

(Δy)² = (0.0001)2 = 0.00000001

so small

Question 47

Describe the impact of yield volatility on the interest rate risk of a bond.

Answer 47

Yield volatility is the standard deviation of the changes in the yield of a bond. Uncertainty about a bond’s future price due to changes in yield results from both a bond’s price sensitivity to yield changes (its duration) and also from the volatility of its yield in the market.

Question 48

Describe credit risk and credit-related risks affecting corporate bonds.

Answer 48

Credit risk refers to the possibility that a borrower fails to make the scheduled interest payments or return of principal. Credit risk is composed of default risk, which is the probability of default and loss severity.

Spread risk is the possibility that a bond loses value because its credit spread widerns relative to its benchmark. Spread risk includes credit migration or downgrade risk and market liquidity risk.

Question 49

Describe seniority rankings of corporate debt and explain the potetntial violation of the priority of claims in a bankruptcy proceeding.

Answer 49

Corporate debt is ranked by seniority or priority of cliams. Secured debt is a direct claim on specific firm assets and has priority over unsecured debt. Secured or unsecured debt may be further ranked as senior or subordinated. Priority of cliams may be summarized as follows:

• First mortgage or first lien
• Second or subsequent lien
• Senior secured debt
• Senior subordinated debt
• Senior unsecured debt
• Subordinated debt
• Junior subordinated debt

Question 50

Distinguish betweeen corporate issuer credit ratings and issue credit rating and describe the rating agency practice of “notching”

Answer 50

Issuer credit ratings, or corporate family ratings, reflect a debt issuer’s overall creditworthiness and typically apply to a firm’s senior unsecured debt.

Issue credit ratings, or corporate credit ratings, reflect the credit risk of a sepcific debt issue. Notching refers to the practice of adjusting an issue credit rating upward or downward from the issuer credit rating to reflect the seniority and other provisions of a debt issue.

Question 51

Explain risks in relying on rating from credit rating agencies

Answer 51

Lenders and bond investors should not rely exclusively on credit rating from rating agencies for the following reasons:

• credit ratings can change during th elife of a debt issue
• rating agencies cannot always judge credit risk accurately
• firms are subject ot risk of unforeseen events that credit ratings do not reflect
• market prices of bonds often adjust more rapidly than credit ratings

Question 52

Explain the components of traditional credit analysis

Answer 52

four c’s of credit analysis:

• capacity - the borrowers abilityy to make timely payment on its debt
• collateral - the value of assets pledged against a debt issue or avilable to creditors if the issuer defaults
• covenants - provisions of a bond issue that protect reditors by requiring or prohibiting actions by an issuer’s management
• character - assessment of an issuer’s management, strategy, quality of earnings, and past treatment of bond holders

Question 53

Calculate and interpret financial ratios used in credit analysis

Answer 53

Credit analysts use profitability, cash flow, and leverage and coverage ratios to assess debt issuer’s capacity

• Profitability refers to operating income and operating profit amrgin, with operating income typically defined as EBIT
• Cash flow may be measured as EBITDA, funds from operations (FFO), free-cash flow before dividends, or free cash flow after dividends
• Leverage ratkos include debt-to-capital, debto-to-EBITDA, and the FFO to debt
• Coverage ratios include EBIT-to-interest expense and EBITDA -to-interest expense
  *

Question 54

A credit analyst is assessing Saxor, a US multimedia company with the following selected financial information:

(figure)

Calculate the cash flows and ratios listed above. Free cash flow is after dividends for all calculations

(figure)

Answer 54

(figure)

Question 55

Coyote Media is alos a multiedia company and is a rival of Saxor. Given the following ratios for Coyote over the same period, calculate the 3-year averages for both Saxor and Coyote and comment on which multimedia company is expected to have a better credit rating.

Answer 55

(figure)

All ratios support a higher credit rating for Saxor. Saxor has a better operating margin and better coverage for interest (EBITDA/interest) and for debt (FCF/dbt). Lower leverage as measured by debt-to-capital and debt-to-EBITDA also favor Saxor.

Question 56

Evaluate the credit quality of a corporate bond issuer and a bond of the issuer, given key financial ratios for the issuer and the industry.

Answer 56

Lower leverage, higher interest coverage, and greater free cash flow imply lower credit risk and a higher credit rating for a firm. When calculating leverage ratios, analysts should include in a firm’s total debt its obligations such as underfunded pensions and off-balance sheet financing.

For a specific debt issue, secured collateral implies lower credit risk compared to unsecured debt and higher seniority implies lower credit risk compared to lower seniority

Question 57

A credit rating agency publishes the following benchmark ratios for bond issues of multimedia companies in each of the investment grade ratings, based on 3-year averages over the period 2011-2013:

(figure)

Based on the ratios calculated in the previous example and the industry standards in the table above, what are the expected issuer credit ratings for Coyote and Saxor?

Answer 57

(figure)

Based on the ratio averages, it is most likely that Saxor’s issuer rating is AA and Coyote’s issuer rating is A.

Question 58

Coyote Media decides to spin off its tevevision division. The new comopany, CoyTV will issue new debt and will not be a restricted subsidiary of Coyote Media. CoyTV is more profitable and generates higher and less volatile cash flows. Describe possible notching for the new CoyTV issue and the potential credit rating change to Coyote Media.

Answer 58

Because CoyTV may be a better credit risk due to a better profit potential, the new issue may have a credit rating one notch above Coyote Media.

Coyote Media may now be less profitable and could have more volatile cash flows. This suggests an increase in credit risk that could lead to a credit rating downgrade.

Question 59

Describe factors that influence the level and volatility of yield spreads.

Answer 59

Corpoarte bond yields comprise the real risk-free rate expected inflation rate, credit spread, maturity premium, and liquidity premium. An issue’s yield spread to its benchmark includes its credit spread and liquidity premium.

The level and volatility of yield spreads are affected by:

• the credit and business cycles
• the performance of financial markets as a whole
• availability of capital from broker-dealers
• supply and demand for debt issues

Yield spreads tend to narrow when

• the credit cycle is improving
• the economy is expanding
• financial market and investor demand for new debt issues are strong

Yield spreads tend to widen when the credit cycle, economy, and financial markets are weakening, and in periods when the supply of new debt issues is heavy or broker-dealer capital is insufficient for market making.

Question 60

Calculate the return impact of spread changes

Answer 60

Analysts can use duration and convexity to estimate the impact on return (the percentage change in bond price) of a change in credit spread.

For small spread changes:

return impact ≈ -duration * Δspread

For larger spread changes:

return impact ≈ -duration * Δspread + 1/2convexity * (Δspread)²

• Make sure convexity is scaled properly. For option-free bonds, convexity should be on the same order of magnitude as modified duration squared. ex. if duration is 6.0 and convexity is 0.562, duration squared is 36.0 and correctly scaled convexity is 56.2*
• Also notice that convexity is divided in half here, but wasn’t when we adjusted for convexity in earlier topic review. This is because differnt authors calculate convexity differently. For the exam, use one-half times convexity if a question asks for the return imapact of a change in spread.*

Question 61

An 8- year semiannual-pay corporate bond with a 5.75% coupon is priced at $108.32. This bond’s duration and reported convexity are 6.4 and 0.5. The bond’s credit spread narrows by 75 basis points due to a credit rating upgrade. Estimate the return impact with and without the convexity adjustment.

Answer 61

return impact (without convexity adjustment)

≈ - modified duration * Δspread

≈ -6.4 * -0.0075

≈ 0.0480

≈0.048 or 4.80%

return on impact with convexity adjustment

≈ - modified duration * Δspread + 1/2 convexity * (Δspread)²

≈ -6.4 * -0.0075 + 1/2(50.0) * (-0.0075)²

≈ 0.0480 + 0.0014

≈ 0.0494 or 4.94%

Notice that convesity needed to be corrected ot mathc the scale of duration

We can caculate the actual change in the bond’s price form the informatin given to illustrate the need for the convexity adjustment

N=16; PMT=5.75/2; FV=100; PV= -108.32; CPT I/Y = 2.25 * 2 = 4.50

YTM after upgrade: 4.50 - 0.75 = 3.75%

Price after upgrade:

I/Y = 3.75 / 2 = 1.875; CPT PV = -113.71

The calculated bond price of $113.71 is an increase of (113.71/108.32) - 1 = 4.98%

The approximation is closer with the convexity adjustment.

Question 62

Explain special considerations when evaluating the credit of high yield, sovereign, and municipal debt issuers and issues.

Answer 62

High yield bonds are more likely to default than investment grade bonds, which increases the importance of estimating loss severity. Analysis of high yield debt should focus on liquidity, projected financial performance, the issuer’s corporate and debt structures, and debt convenants.

Credit risk of sovern debt includes the issuing country’s ability and willingness to pay. Ability to pay is greater for debt issued in the country’s own currency than for debt structures, and debt covenants.

Analysis of general obligation municipal debt is similar to analysis of sovereign debt, focusing on the strngth of the local economy and its effect on tax revenues. Analysis of municipal revenue bonds is similar to analysis of corporate debt, focusing on the ability of a project to generate sufficient revenue to service the bonds.

Question 63

Two European high yield companies in the same industry have the following financial information:

(figure)

1. calculate total leverage through each level of debt for both companies
2. calculate net leverage for both companies
3. comment on which company is more attractive to an unsecured debt investor

Answer 63

(figure)

Company B has a lower secured debt leverage ratio than Company A, while total and net leverage ratios are about the same. Comapny B is more attractive to unsecured debt holders because it is less top heavy and may have some capacity to borrow from banks, which suggest a lower probability of default. If it does default, Company B may have a higher percentage of assets available to unsecured debt holders than Company A, especially if holders of convertible bonds have exercised their options.