Equations

Question 1

Give the equation for the force between molecules (F) and annotate.

Answer 1

F = (-Z₁Z₂)÷4πe_or²

F: a vector, direction depends on the sign of charges:

• charges the same is -ve
• opposite charges +ve

Z: charges on ions

e_o: permitivity of free space (8.8542 x 10^-12 Fm^-1)

r²: separation of charges

Question 2

Give the equation for work done for a force moving a small distance (dr) and define U.

Answer 2

work = dU = F dr

U = (Z₁Z₂)÷(4πe_or)

Values of U:

• 0 for infinite separation.
• -ve for attractive forces
• +ve for repulsive forces

Question 3

Give the equation for Coulombic energy.

Answer 3

Ø_c = (-|Z₊||Z_-|e²) ÷ (4πe_or’)

• Ø_c = 0 when r = infinity.
• Ø_c = -ve for ions of opposite polarity.
• Ø_c = +ve for ions of like polarity - change the sign at the front of the equation.

Question 4

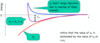
Draw the potential energy diagram for two ions of opposite polarity, showing all energy lines + equation.

Answer 4

ø_r: short range repulsion, a balancing repulsive force that prevents the ions collapsing to 0 distance.

ø_c: Coulomb energy

ø_T = ø_c + ø_r

ø_T = [(- |Z₊| |Z_-| e²) ÷ 4πe_or] + b e^(-r/p)

when r = r_o, the ions are at their equilibrium separation.

Question 5

Give the equation for the lattice energy (U_L) of one ion.

Answer 5

Ø_c = [(-|Z₊||Z_-|e²)÷4πe_or] x A + b e^(-r/p)

A: Madelung constant

+ term: the repulsive term to get the total energy of one ion with all the other ions in the crystal.

Question 6

Give the equation for the lattice energy (U_L) for two ions.

Answer 6

U_L = -ø_T = [(A N_A |Z₊||Z_-]e²)÷(4πe_or_o)] x [1-p/r_o]

p: 34.5 pm for most alkali halides

r_o: the radii of the anion + cation added together

Question 7

Give Kapustinski’s equation + outline when we would use it.

Answer 7

U_L = (1.214 x 10⁵ V |Z₊||Z_-|)÷(r₊ + r_-) [1 - p/r₊ + r_-]

Used when we only have the formula for an ionic solid but don’t know the structure or Madelung constant.

Question 8

Give the equation for thermogravimetric analysis.

Answer 8

Question 9

Draw the graph for differential thermal analysis and give the equation for the energy involved in the phase change.

Answer 9

energy (J) = power (Js^-1) x time (s)

Question 10

Draw a primitive unit cell.

Answer 10

Question 11

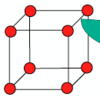
Draw a body centred cubic (BCC) unit cell.

Answer 11

Question 12

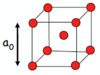
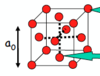
Draw a face centred cubic (FCC)/cubic close packed (CCP) unit cell.

Answer 12

Question 13

Draw the primitive and side-centred orthorhombic bravais lattice.

Answer 13

Question 14

Draw the 4 unit cells of an orthorhombic system.

Answer 14

Question 15

Give the equation for the energy of electrons.

Answer 15

E = h² ÷ (2mi²)

• m: mass of electron
• i\ = wavelength
• E ~ 37.6 eV

Question 16

Give the equation for the energy of a neutron.

Answer 16

E = h² ÷ (2mi²) = p²÷2m = 1/2 mv²

• The energy for neutrons is so small we talk aboout the speed v.
• v ~ 1979 ms^-1 if a gas at RT.

Question 17

Give the Bragg equation.

Answer 17

ni\ = 2dsin*theta*

• n: order of diffraction
• i: x-ray wavelength
• d: distance between layers
• *theta*: angle of incidence + diffraction

Question 18

Give the equation for the separation d_{(h, k, l)} between planes (h k l) for a cubic system.

Answer 18

d_{(h, k, l)} = a ÷ (h² + k² + l²)^1/2

• a: length of side of the unit cell cube