EM Summary deck

Question 1

Lorentz force on a moving charge

Answer 1

Question 2

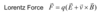
What does this represent

Answer 2

magnetic flux density

Question 3

What are these two?

Answer 3

electric permittivity

magnetic permeability

Question 4

State Gauss’s Law (electric field) and its respective equation

Answer 4

For the electric field: The total electric flux (or flow of the vector D) across a closed

surface Ω, enclosing a volume V is equal to the total electric charge contained in V.

Question 5

State Gauss’s Law (magnetic field) and its respective equation

Answer 5

For the magnetic field: There is no magnetic charges, so the total magnetic flux (or flow of the vector B) across a closed surface Ω, enclosing a volume V is equal to zero.

Question 6

State Faradays Law and equation

Answer 6

Faraday’s Law: “A time-varying magnetic field generates a circulating electric field”.

Question 7

State Ampere’s Law and equation

Answer 7

“An electric current generates an magnetic field circulating around it.”

Question 8

Continuity Condition - conservation of charge

Answer 8

Question 9

Time-Harmonic fields representation

Answer 9

Question 10

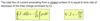
Constitutive Relations

Answer 10

Question 11

Boundary Conditions: Conditions at a dielectric interface

Answer 11

Question 12

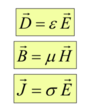
Boundary Conditions: Conditions at a perfectly conducting surface

Answer 12

Question 13

Solution of Wave Equation in Free Space: Plane Waves

Answer 13

Question 14

Phase Velocity, Group Velocity and Wavelength of Plane Wave in free space

Answer 14

Question 15

Phase Velocity, Group Velocity andWavelength of Plane Wave in a medium ε

Answer 15

Question 16

Plane Wave expression for waves in both directions

Assuming propagation along z axis

Answer 16

Question 17

Polarizaiton: Linear, Circular and Elliptical

Answer 17

Question 18

What does the Poynting Vector Represent?

If you take the integral of the Poyting wrt surface Ω, what do you get?

Answer 18

Instantaneous power flow density

Total instantaneous power flow across a surface Ω

Question 19

What does the complex Poynting vector represent?

and its integral over a surface Ω?

Answer 19

Time-average power density

(note the lack of the term ‘flow’ compared to regular Poynting)

Total time-average power flow across a surface Ω

Question 20

Reflection and Refraction: What are Snell’s Laws ?

Answer 20

So the reflected angle is equal to the incident angle.

note that the square root of the relative permittivity gives the refractive index.

Question 21

Reflection and Refraction: What does parallel polarization entail?

Answer 21

Electric field in plane of incidence

i.e. parallel to the incidence

Question 22

Reflection and Refraction: What does perpendicular polarization entail?

Answer 22

Electric field normal to the plane of incidence

Question 23

What is Brewsters Angle?

Answer 23

For the case of parallel polarisation, there is an angle of incidence, the Brewster angle for which there is no reflection.

Question 24

What are the reflection and transmission coefficients for a normal incidence of a plane wave on a medium?

Answer 24

Question 25

Wave propagation in Conductive Media: Equation for effective permittivity?

Answer 25

Question 26

Wave propagation in Conductive Media: What is the propagation constant given as?

Answer 26

Question 27

Wave propagation in Conductive Media: the relation between propagation constant and E?

Answer 27

Question 28

Wave propagation in Conductive Media: What are good conductors defined by?

Answer 28

Question 29

Reflection and Transmission through Conductors: (good conductor)

Answer 29

Question 30

Waveguides: The TEM Mode

Answer 30

Question 31

Waveguides: The TE and TM Mode

Answer 31

Question 32

Waveguides: Hollow Pipe Waveguide

Answer 32

Question 33

Waveguides: Rectangular Waveguide The Modes

Answer 33

Question 34

Waveguides: The dominant mode TE₁₀

Answer 34

Question 35

What does taking the divergence of an integral do...

Answer 35

give you the argument inside the integral