Congruences Flashcards
How do you write a is congruent to b, mod n?
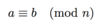
When do we write
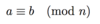

What is a residue class?
We group a and b from the following into the same class.

How do you denote a residue class?

What are the residue classes for n=3?
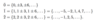
Finish the following proposition.


Prove the following proposition.


Can you divide congruences?
No
Finish the following proposition.
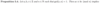
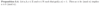
Prove the following proposition.


Define the complete set of residues.
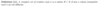
Finish the following lemma.
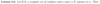

Prove the following lemma.


What is a linear congruence?

Prove the following proposition.
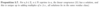

What is the method for solving linear congruences of the form ax ≡ b (mod n)?
Where :-) equals ax ≡ b (mod n)

Finish the following proposition.

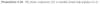
Prove the following proposition.


What is the name of the theorem that can be used to solve linear systems?
Chineese remainder theorem.
What is the chineese remainder theorem?

Prove the following theorem.


Define Euler’s φ-function.

What is another way to think of Euler’s φ-function?
In other words, it is the number of positive numbers below n which are coprime to n.
What is Euler’s theorem?