Chapter 2: Reflection of Light Flashcards
What are the 2 laws which govern reflection of light at any interface?
- The incident ray, reflected ray and normal to reflecting surface all lie in the same plane
- Angle of incidence, i, equals the angle of reflection, r.
Why would a perfect reflecting surface be invisible?
when parallel light encounters an irregular surface it is scattered in many directions (diffuse relfection)
this is how most objects (that aren’t self-luminous) are seen
What are the 5 properties of the images of objects formed by reflection at a plane surface (e.g. flat mirror)?
- virtual
- erect
- laterally inverted
- lie along line perpendicular to the reflecting surface
- are as far behind the surface as the object is in front of it
What happens to the reflected ray from a mirror if the plane mirror is rotated?
the reflected ray is deviated through an angle twice the angle of rotation of the mirror

What are the 4 key features of concave and convex mirrors to be aware of when drawing ray diagrams?
- centre of curvature, C, is centre of sphere of which the mirror is a part
- pole of the mirror, P, is centre of the reflecting surface
- Principle focus, F is where rays parallel to the principal axis are reflected towards (concave) or away from (convex)
- CP is twice the length of FP
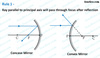
Are the images of objects an infinite distance away on the principal axis real or virtual, for concave and convex spherical mirrors?
- concave = real
- convex = virtual
will fall on principal focus in both cases (F)
What are the 2 rays you need to construct when determining the image from a spherical mirror?
- line from top of object that is paralell to the principal axis, and is reflected to or away from F
- line from top of object that goes straight through C and is reflected back in the same direction
What + where will the image be for a concave mirror + object outside the centre of curvature, C?
real, inverted, diminished, between C and F
What + where will the image be for a concave mirror + object between the centre of curvature, C and principal focus, F?
real, inverted, enlarged, lying outside centre of curvature C
What + where will the image be for a concave mirror + object inside the principal focus, F?
erect, virtual, enlarged (rays travelling away from the mirror are diverging, but convergent on the other side therefore virtual image)
What + where will the image be for a convex mirror at any distance from the mirror?
virtual, erect, diminished
How can you calculate the position of the image formed by a spherical mirror?
you need the focal length and the distance of the object from the mirror:
1/v - 1/u = 1/f (or 2/r)
where v = distance of image from mirror, u = distance of object from mirror, f= focal length, r= radius of curvature
How can you calculate magnification of a spherical mirror?
i/o = -v/u
i=image size, o=object size, v=distance of image from mirror, u=distance of object from mirror


