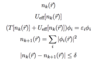Chapter 12: Quantum Calculations

Question 1

Hamiltonian under Born-Oppenheimer Approximation

Answer 1

• can separate and decouple nuclear and electronic wavefunctions

Question 2

Hartree Method

(Assumptions)

Answer 2

• non-interacting electrons

Question 3

Hartree Wavefunction

Answer 3

• Drawbacks:
  
  • not antisymmetric → no exchange
  • only include Coulomb correlation

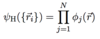
Question 4

Hartree Single Particle Equation

Answer 4

• gives energy and orbital for electron i

Question 5

Hartree-Fock Wavefunction

Answer 5

• uses Slater determinant to form wavefunction
  
  • includes Pauli Principle exchange

Question 6

Hartree-Fock Single Particle Equation

Answer 6

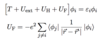
Question 7

Hartree-Fock Approximations

Answer 7

• Born-Oppenheimer
• Coulomb correlation only
• non-relativistic
• attainable phase space is constrained by single Slater determinant

Question 8

Post-HF Methods

Answer 8

• mostly aim to increase correlation
• may try to increase phase space
• e.g. Coupled-Cluster Method

Question 9

Coupled-Cluster Method

Answer 9

• can look at excited states
• good for chemical reactions with small molecules
• increases correlation

Question 10

Hartree-Fock Limit

Answer 10

• HF groundstate prediction be bounded by below and is an overestimate for true groudstate

Question 11

Density Functional Theory

Answer 11

Reduces 3N-dimensional wavefunction to 3-dimensional wavefunction using density n(r )

Question 12

Hohenberg-Kohn Theorems

Answer 12

1. There exists a one-to-one mapping between groundstates density and groundstate wavefunction for non-degenerate groundstates
2. Groundstate density minimizes total energy.

Question 13

Kohn-Sham Equation

Answer 13

• ε_<em>i</em>, φ_i are for fictitious particle i
• however, densities match n_DFT(r ) = n_exact(r )

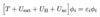
Question 14

Exchange-Correlation Functional

Answer 14

Question 15

Local Density Approximation

Answer 15

• local functional
• uses homogeneous electron gas exchange-correlation
• fails for vdW forces and metallic systems

Question 16

Generalized Gradient Apprixmation

Answer 16

• semi-local functional
• also depends on gradient of density

Question 17

DFT Short-Comings

Answer 17

• overbinding → underestimates bandgap
• can only predict trends for excited states → TDDFT better for excited states

Question 18

Time-Dependent DFT

Answer 18

• rewrite KS equations in time-dependent manner using quantum mechanical action A[n]
• need new functionals because (non-)locality can occur in space and time

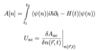
Question 19

Pseudo-Potentials

Answer 19

Used to neglect core electrons and avoid singularities

• functional specific

Question 20

DFT Algorithm

Answer 20

1. Initial guess of density
2. Evaluate potential
3. Solve KS equation
4. Get new density
5. Test for self-consistency