Booklet 3 - Electricity (Worked Examples) Flashcards
Use the oscilloscope trace to calculate
(a) the frequency
(b) the peak voltage

(a) The distance between crests is 4 cm.
The time base is set at 5 ms cm–1
The period of the wave is
T = 4 x 5ms = 4 0.005s = 0.02 s
f = 1/T
f = 1/0.02
f = 50 Hz
(b) The height from bottom to top is 8 cm.
The height from middle to top is ½ × 8 = 4 cm
The volts/div was set at 2 V cm–1,
The peak voltage is:
Vpeak = 4 × 2
Vpeak = 8 V
A power pack is labelled with a voltage of 12 V r.m.s.
Calculate the peak voltage.
Vpeak = √2 x Vrms
Vpeak = 1.41 x 12
= 17.0 V
Example
Calculate the current flowing during a bolt of lightning which lasts for 10 ms and transfers 50 C of charge.
Q = 50 C
I = ?
t = 10 ms = 10 x 10-3 s
Q = It
50 = I x 10 x 10-3
I x 10 x 10-3 = 50
I = 50/10 x 10-3
I = 5000 A
Example
Calculate the current flowing when a 2 kΩ resistor is connected to a 6V battery.
V = 6 V
I = ?
R = 2 kΩ = 2 x 103 Ω
V = IR
6 = I x 2 x 103
I x 2 x 103 = 6
I = 6/2 x 103
I = 3 x 10-3 A
Example
Calculate the current drawn when a 2 kW kettle is plugged into the mains.
P = 2 kW = 2000 W I = ? V = 230 V (UK Mains Voltage - see previous LO)
P = IV
2000 = I x 230
I x 230 = 2000
I = 2000/230
I = 8.70 A (to 3 sig fig)
Example
Calculate the current flowing when 3500 W of power are supplied to a 100 Ω resistor.
P = 3500 W I = ? R = 100 Ω
P = I2R
3500 = I2 x 100
I2 x 100 = 3500
I2 = 3500/100
I2 = 35
I = √35
I = 5.92 A (to 3 sig fig)
Example
A 100 Ω resistor is attached to a 9 V battery. Calculate the power supplied.
P = ? V = 9 V R = 100 Ω
P = V<sup>2</sup>/R P = 9<sup>2</sup>/100 P = 81/100 P = 0·81 W
Example
Calculate the total resistance of the circuit shown.
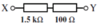
R<sub>T</sub> = ? R<sub>1</sub> = 1·5 kΩ = 1500 Ω R<sub>2</sub> = 100 Ω
R<sub>T</sub> = R<sub>1 </sub>+ R<sub>2 </sub>+ .... R<sub>T</sub> = 1500 + 100 R<sub>T</sub> = 1600 Ω

Example
Calculate the total resistance of the circuit shown.
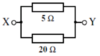
RT = ? R1 = 5 Ω R2 = 20 Ω
1/R<sub>T</sub> = 1/R<sub>1 </sub>+ 1/R<sub>2 </sub>+ .... 1/R<sub>T</sub> = 1/5 + 1/20 1/R<sub>T</sub> = 1/4 R<sub>T</sub> = 4/1 = 4 Ω
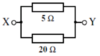
Example
Calculate the total resistance of the circuit shown.

25 & 25 in series
RT = ? R1 = 25 Ω R2 = 25 Ω
R<sub>T</sub> = R<sub>1 </sub>+ R<sub>2 </sub>+ .... R<sub>T</sub> = 25 + 25 R<sub>T</sub> = 50 Ω
50 (above) in parallel with 100
RT = ? R1 = 50 Ω R2 = 100 Ω
1/R<sub>T</sub> = 1/R<sub>1 </sub>+ 1/R<sub>2 </sub>+ .... 1/R<sub>T</sub> = 1/50 + 1/100 1/R<sub>T</sub> = 3/100 R<sub>T</sub> = 100/3 = 33.3 Ω (to 3 sig fig)

A cell of emf 1.5 V is connected in series with a 28 Ω resistor. A voltmeter measures the
voltage across the cell as 1.4 V.
Calculate:
(a) the internal resistance of the cell
(b) the current if the cell terminals are short circuited
(c) the lost volts if the external resistance R is increased to 58 Ω.

(a) E = 1.5 V
R = 28 Ω
V = 1.4 V
In this case we do not know the current, I, but we can work it out:
V = IR
1.4 = I x 28
I = 0.05 A
E = V + Ir 1.5 = 1.4 + 0.05 x r r = 2 Ω
(b) Short circuit: R = 0, V = 0; Assume E (1.5 V) and r (2 Ω) constant
E = V + Ir
1.5 = 0 + I x 2
I = 0.75 A
(c) Assume E (1.5 V) and r (2 Ω) constant
Combine E = V + Ir and V = IR to get E = I(R + r)
1.5 = I x (58 + 2)
I = 1.5/60 = 0.025 A
lost volts = Ir
= 0.025 × 2
= 0.05 V
Example
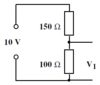
Calculate V1 in this circuit.
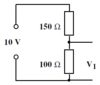
V<sub>1</sub> = ? R<sub>1</sub> = 100 Ω (This is R<sub>1</sub> because it has been marked as V<sub>1</sub> in the question) R<sub>2</sub> = 150 Ω V<sub>s</sub> = 10 V
V<sub>1</sub> = R<sub>1</sub>/(R<sub>1</sub>+R<sub>2</sub>) x V<sub>s</sub> V<sub>1</sub> = 100/(100+150) x 10 V<sub>1</sub> = 4 V
(NB The formula sheet uses V2 = R2/(R1+R2) x Vs but this can be adapted as above as long as the voltage and resistor in bold agree with each other i.e.
V1 = R1/(R1+R2) x Vs
or
V2 = R2/(R1+R2) x Vs
The choice of which resistor is labelled 1 or 2 doesn’t matter unless it has already been marked in the Q)
Example
Calculate V1 in this circuit.
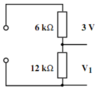
V1 = ?
V2 = 3 V
R1 = 12 kΩ
R2 = 6 kΩ
(Can leave R1 and R2 in kΩ since the formula is a ratio.)
V<sub>1</sub>/V<sub>2</sub> = R<sub>1</sub>/R<sub>2</sub> V<sub>1</sub>/3 = 12/6 V<sub>1</sub> = 3x12/6 V<sub>1</sub> = 6 V

A circuit is attached to XY which has a resistance of 10 kΩ. Calculate the effective
resistance between XY and thus the voltage across XY.

The resistance between XY comes from the two identical 10 kΩ resistors in parallel.
The combined resistance is therefore 5 kΩ (You can check this using 1/RT = 1/R1 + 1/R2).
Use this resistance as R1 in the potential divider formula.
R1 = 5 kΩ
R2 = 20 kΩ
VS = 12 V
V1 = ?
V<sub>1</sub> = R<sub>1</sub>/(R<sub>1</sub> + R<sub>2</sub>) x V<sub>s</sub> V<sub>1</sub> = 5/(5 + 20) x 12 V<sub>1</sub> = 2.4 V
A capacitor stores 4 x 10-4 C of charge when the potential difference across it is 100 V.
(a) Calculate the capacitance.
(b) Calculate the energy stored in the capacitor.
C = ? Q = 4 x 10<sup>-4</sup> C V = 100 V
(a) C = Q/V
= 4 x 10-4/100
= 4 x 10-6 F
(b) E = ½QV
E = ½ x 4 x 10-4 x 100
E = 0.02 J
A 40 µF capacitor is fully charged using a 50 V supply.
Calculate the energy stored in the capacitor.
E = ? C = 40 µF = 40 x 10<sup>-6</sup> F V = 50 V
E = ½ CV<sup>2</sup> E = ½ x 40 x 10<sup>-6</sup> x 50<sup>2</sup> E = 0.05 J
A 50 µF capacitor stores 2 mC of charge when fully charged.
Calculate the energy stored in the capacitor.
E = ? Q = 2 mC = 2 x 10<sup>-3</sup> C C = 50 µF = 50 x 10<sup>-6</sup> F
E = ½ Q<sup>2</sup>/C E = ½ x (2 x 10<sup>-3</sup>)<sup>2</sup> / 50 x 10<sup>-6</sup> E = 0.04 J


