Attenuation & Decibels Flashcards
___ is How the strength of the ultrasound changes as is moves through the medium
attenuation
____ is the Rate that work is done, energy
in US it is a wave of energy
Some energy is released into the medium during propagation
Measured in _____
Determined by the _____
power
Watts (mW)
source
_____ is the Power of the wave per area over which it is spread. what is it determined by?
intensity
source
what is the formula for intensity? what is it measured in?
I (W/cm2) = Power (W)/ Area (cm2)
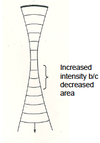
what is intensities relationship to power and area?
Increasing power increasing Intensity
Decreasing area increasing Intensity - focusing
Example: Sun on dry leave, focused through a lens will increase the intensity and cause leaves to burn – power is unchanged, only area changed
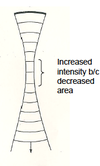
what increases w/ increasing power and intensity?
what improves the image quality?
potential bioeffets.
Image quality – Will generally be improved by increasing power because you get stronger reflections back
_____ is the Maximum variation of an acoustic variable
Unit depends on the variable being measured
amplitude
what is the relationsihp b/t amplitude and intensity?
Intensity = amplitude2
Amplitude = square root of Intensity
If amplitude increased by 2x, then intensity increased 4x
what are power and attenuation described in ?
decibels
what is a decibel?
what does it represent?
what do you need to compute a decibel change? why?
Bel – logarithmic ratio of the relative powers of 2 beams.
Decibel is 1/10 of a bel, it is a unit of measurement used in sound applications
Represents the smallest degree of difference in loudness the normal human ear can hear
Relative values, not absolute – need 2 powers or intensities to compare in order to compute a decibel change
*50% off of $100 = $50 it has changed by 50$. if it’s 3 decibels, it has increased 2x from where it started
what is a logarithms
Different way to look at numbers, called log base 10
The log of a number is the amount of times 10 has to multiplied together to get the number.
Also described as the power to which 10 must be raised to get the number
Look at the chart
what is
Log10 of 10 =
Log10 of 100 =
Log10 of 1000 =
Log of a fraction is –
Log10 of .01 = -2
why are these used?
1
2
3
Logs are used when dealing with very large numbers (to many 0). In sound application we are comparing 2 like quantities, such as power, intensity or amplitude
When comparing power, intensity or amplitude change
if there is an increase, it is a ____
If there is a decrease, it is an _________
gain
attenuation
Input power is 10W, output power is 100W. What is the decibel change? Is it a gain or attenuation?
Input power is 100W, output power is 10W. What is the decibel change? Is it a gain or attenuation? What is the power ratio?
Beginning intensity is 100 mW/cm2, ending intensity is 10 mW/cm2. What is the decibel change? Is it a gain or attenuation? What is the intensity ratio?
Beginning intensity is 100 W/cm2, ending intensity is 10 mW/cm2. What is the decibel change? Is it a gain or attenuation? What is the intensity ratio?
Units must match.
When comparing voltage and amplitude, you must use ___in the equation
Because intensity = amplitude2
Because Power = voltage2
20
Beginning voltage is 100 mV, ending voltage is 10 mV. What is the decibel change? Is it a gain or attenuation? What is the voltage ratio?
The input intensity is 10 times less than the output intensity. What is the intensity ratio? What is the “relative intensity?”
This is asking for dB change.
The transmitted intensity is 1000 times greater than the reflected intensity. What is the relative intensity?
This is asking for dB change.
Power one is 100 W and power two is 50 W. What is the decibel change?
Power one is 100 W and power two is 200 W. What is the decibel change?
what are the intensity power ratios for 3, 6, 10
Power one is 100 W and power two is 25 W. What is the decibel change?
Power one is 100 W and power two is 400 W. What is the decibel change?
3 dB change is increasing quantity__times
-3 dB change is decreasing quantity to __
6 dB change is increasing quantity __times
-6 dB change is decreasing quantity to ___
10 dB change is increasing quantity____ times
-10 dB change is decreasing quantity to 1____
20 dB change is increasing quantity ___ times
-20 dB change is decreasing quantity to ___
3 dB change is increasing quantity 2 times
-3 dB change is decreasing quantity to ½ (.5)
6 dB change is increasing quantity 4 times
-6 dB change is decreasing quantity to ¼ of original (.25)
10 dB change is increasing quantity 10 times
-10 dB change is decreasing quantity to 1/10 of original (.1)
20 dB change is increasing quantity 100 times
-20 dB change is decreasing quantity to 1/100 of original (.01)
what are the casues of attenuation?
(RR SAID)
Absorption (conversion to heat)
Reflection
Scatter
Interference
Refraction
Diffraction
what does the amnt of attenuation depend on ?
how is it measured?
Medium, Frequency
Measured in decibels (dB)
____ is Secondary to internal friction of molecular vibration.. it Converts US energy to heat
what is it’s relationship to frequency?
absorption
higher frequency, increased absorption
____ is affected by
Viscosity – related to adhesiveness of molecules in medium. Increased viscosity increased absorption
Relaxation time – time required for molecules to return to equalibrium position after wave passed. Increased relaxation time, increased absorption
absorption
____ is When 2 waves combined together
Combinations of positive and/or negative pressures (Kremkau)
Variation with distance or time of the amplitude of a wave, which results from the addition of two or more waves (Zagzebski)
interference
what are the 3 types of interference?
Constructive – combine in phase, make greater amplitude wave
Destructive – combine out of phase, decrease amplitude of wave
Combination – complex wave formed when waves of different frequencies combine
____ is 2 waves with same frequency
Combine in-phase, they interfere constructively and the result has twice the amplitude of the individual waves.
constructive interference
___ is 2 waves with same frequency
When the two waves have opposite-phase (180o out of phase), they interfere destructively and cancel each other out
destructive interference
___ is Wave with different frequencies
Combine to form a complex wave
complex interference
___ is the Spreading out of the beam as it moves away from the source.
when it increases what happens to intensity?
area?
Increasing diffraction causes loss of intensity.
Area becomes larger
Beginning intensity is 100 mW/cm2
End intensity is 50 mW/cm2
What is the dB change?
What is the intensity ratio?
IE = II x Intenisty ratio
- Calculate attenuation coefficient
- Calculate total attenuation
- Find intensity ratio for dB of attenuation
- IE = II x Intenisty ratio
____ is a numerical value used to express how different materials attenuate sound.
what is its unit?
what is it affected by?
Attenuation coefficient
dB/cm
Frequency and Tissue
wht is the attenuation coefficient for soft tissue?
what is its formula?
what happens to it if frequency increases?
Rule of thumb for soft tissue…
Ac = .5 dB/cm for each MHz of frequency
Ac (dB/cm) = .5 dB/cm x F (MHz)
1 MHz transducer will attenuate .5 dB/cm
2 MHz transducer will attenuate 1 dB/cm
Increase frequency, increase Ac
what is the formula for attenuation?
Attenuation (dB) = Ac (dB/cm) x distance (cm)
we can calculate total attenuation by knowing Ac and the distance traveled in the medium
If Ac is 3 dB/cm and the sound travels 1 cm, what is the amount of attenuation?
Attenuation (dB) = Ac (dB/cm) x distance (cm)
3 dB
Using a 4MHz transducer in soft tissue, what is the amount of attenuation after 3 cm of travel?
Ac (dB/cm) = .5 dB/cm x F
Attenuation (dB) = Ac (dB/cm) x distance (cm)
6dB
Once we know attenuation in dB, determine by using the chart what the decimal value (Intensity ratio). so…
3dB attenuation =
6 dB attenuation =
10 dB attenuation =
20 dB attenuation =
3dB attenuation = .5
6 dB attenuation = .25
10 dB attenuation = .1
20 dB attenuation = .01
what is the formula for end intensity?
End intensity = Initial intensity x Intensity ratio
What is the intensity of a 100 W/cm2 , 2 MHz beam after traveling through 10 cm of soft tissue?
what is 1/2 intensity depth formula?
Depth at which the intensity is decreased by ½ (3dB)
½ Int Depth (cm) = 3(dB) / Ac(dB/cm)
Not a new equation, same only rearranged
what is ½ Intensity depth is also known as:
What is the depth at which half intensity occurs in soft tissue for a 4 MHz transducer?
Depth of penetration
½ value layer thickness
½ boundary layer
