5: Radians Flashcards
1
Q
Define “radian”
A
The angle subtended at the centre of a circle by an arc with length equal to the radius.
2
Q
Given that the sector of a circle has angle θ, derive a formula for the:
- arc length
- area
A
Arc length
L = θ/360 x 180/𝜋 x 2𝜋r
L = rθ
Area
A = θ/360 x 180/𝜋 x 𝜋r2
A = 1/2r2θ
3
Q
Give the formulae for the arc length and area of a sector with angle θ.
A
L = rθ
A = 1/2r2θ
4
Q
Give the formula for the area of a triangle within a sector.
A
A = ½r2sinθ
5
Q
Marking a triangle within a sector creates a segment. What is the area of this segment, assuming angle θ?
A
A = ½r2θ - ½r2sinθ
= ½r2(θ - sinθ)
6
Q
Use trig graphs to work out small angle approximations for y = sinθ, y = cosθ and y = tanθ.
A
7
Q
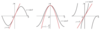
The % error for a value of θ is 1%. Show that 100θ = 101sinθ.
A
