2012 Exam

Question 1

How does OFDM simplify the process of high data rate transmission + reception in a highly time dispersive channel? (4)

Answer 1

• High bit rate single carrier transmission is difficult to achieve
  
  • Time delay spread introduces ISI = BER
• OFDM data is multiplexed over a large # sub-carriers
• Data on each sub-carrier is low enough to avoid ISI
• A FDE uses a complex multiplier to correct the ampl. + phase distortion of each sub-carrier

Question 2

Method for signal processing in an OFDM system to make a cyclical process (2)

Answer 2

Extend the data vector

Resolve the extended lin. conv, it can appear cyclical

Question 3

Processing Gain Definition

Answer 3

Ratio of the Spread Spectrum b/w to message signal

Question 4

DS-SS Definition

Answer 4

take narrowband message + convert to wideband via directly modulating w/ wideband spreading sequence.

Synchronised de-spreading is used to recover the narrowband message

Question 5

Spreading Sequences used in DS-SS? Explain why an arbitrary selection of code can not be used for multi-user networks (3)

Answer 5

• Pseudo Random Binary Sequences (m-sequences)
• Sharp auto-correlation + pseudo-random (Gaussian like) run length distr. of 1’s + 0’s
• Arbitrary selection of maximal length shift register sequences can give rise to very poor cross-correlation + inhibit code separation

Question 6

Describe rank deficient, in terms of cap. + why? (2)

Suggest solⁿ

Answer 6

• Due to correlation in composite antenna + propgating medium, not alltheorthogonalspatial modes are available for thearray dimension exciting the channel
• Reduce the potential spatial multiple gain + channel cap.

Solⁿ - use a wider spaced antenna / polarisation

Question 7

Structure of LTE (2)

Answer 7

• i/p data is passed through the filter memory (feedforward filter) + multiplied w/ appropriate weight
• An error term is generated on a symbol by symbol basis based on the difference between software filter o/p +
  
  • ​training symbol
  • ​hard decision o/p

Question 8

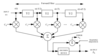
Markov Process

Answer 8

next state is defined by the previous state + current i/p

Question 9

Viterb Eq.

Answer 9

state is defined by data stored in channel memory. Next state is then based on the previous state + i/p symbol (Markov Process)