08 Forces, movement and shape- 4th Form Flashcards
If spring A is a single spring, spring B must be..
1) stiffer spring
2) weaker spring
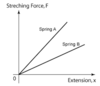
Answer: 2) weaker spring
B has a smaller gradient
It requires less force to stretch by the same amount.
Why is a double decker bus difficult to tip?
It has a low centre of gravity
- The weight acts from the centre of gravity.*
- It will not tip until the weight goes beyond the base*
Below is a velocity-time graph for a parachute jump.
What portion/s of the graph represents the constant speed? How do yoy know?
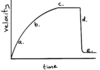
portion c and e
The velocity is constant. The graph is horizontal at c and e
gradient is zero = zero acceleration or constant speed
The parachutist has reached terminal velocity at c and again at e (after he opens his parachute)
How do you find the centre of gravity for a symmetrical object?
Draw lines of symmetry to find the centre of the object.
Below is a force extension graph for a wire. Does the wire obey Hook’e Law?
The wire obeys Hooke’s Law during the straight part of the graph. If the force were removed, the wire behaves elastically and would go back to its original length.
For larger forces the wire no longer obeys Hooke’s Law, the wire behaves plastically and will not go back to its original length.
Define thinking distance
Thinking distance is the distance a car travel after you see the hazard to the point you put your foot on the brake - the distance travelled while you are reacting to the hazard
Below is a velocity-time graph for a parachute jump.
What portion/s of the graph represents the greatest deceleration?
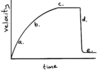
portion d
The velocity is decreasing at d and the gradient is very steep representing a very large deceleration.
Why does the swinging basket finally come to rest?
Swung to one side, the line of the weight (the force) does not act through the pivot at the top of the hanger. M= F x d, there is a moment about the pivot making it swing.
When at rest the line of of the weight (the force) acts through the pivot at the top of the hanger. M = F x d. there is no moment about the pivot. No longer swings.
What is the Centre of Gravity?
The point on an object where the weight appears to act.
If one spring needs 5 N to stretch 10 cm. How far would this spring system stretch with 5 N pulling on it.
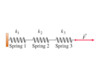
There are three springs in the series system. Each spring will stretch 10 cm. The total stretch of the series spring system would be 30 cm.
What factors affect thinking distance of a vehicle?
tiredness
drinking or drug taking
speed of vehicle
distractions such as mobile phone
Define braking distance
The distance a vehicle travels while the brakes are applied and the vehicle comes to rest.
How can the elephant balance on the ball?
The centre of gravtiy of the elephant is located over the ball
The weight of the elephant passes through the ball (base)
There is no perpendiular distance to the pivot and no moment (turning effect)
How do you find the stopping distance from thinking distance and braking distance?
stopping distance = thinking distance + braking distance
If the speed of a vehicle doubles, what happens to the braking distance?
As speed double the braking distance more than doubles- it actually quadruples!
If the single spring needs 2 N to stretch 10 cm, how many centimetres will the series spring system stretch with the same force?
There are two springs in series with 2 N hanging on them. They will both stretch by 10 cm.
The overall stretch will be 20 cm.
Which spring is stiffer or has the greater spring constant?
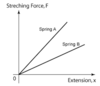
Spring A needs more force to stretch the same distance as spring B.
Gradient for spring A is greater.
What is the equation that links moment, force and perpendicular distance?
Moment = force x perpendicular distance
What factors affect braking distance of a vehicle?
tread depth of tyres
icy or wet roads
mass of vehicle
condition of brakes
speed of vehicle
What is the principle of moments?
For an object to be in equilibrium
sum of the clockwise moments must equal the sum of the anticlockwise moments
What is the relationship between weight, mass and acceleration?
W = mg
Weight = mass x acceleration
Which spring is stiffer or has the greater spring constant?
Spring A needs more force to stretch the same distance as spring B.
Gradient for spring A is greater.
Below is a force vs length graph for a spring.
a) What is the original length of the spring?
b) What is the extension at 4 N?
Read the length at 0 N
Therefore the original length is 17.5 cm
extension = length - original length.
extension = 40.0 - 17.5 = 22.5 cm
How do you find the centre of gravity of a lamina?
1- punch three holes along edge of the lamina
- hang the lamina on a horizontal pin in a cork
- hang plumbline infront of lamina
- let the lamina and plumbline come to rest
- mark plumbline position down the lamina
- repeat for other two holes.
- centre of gravity is located where all three lines cross.
What will happen to an object if its total anticlockwise moments do not equal the total clockwise moments?
The object will turn
Moment is a vector quantitiy and there will be a resultant moment
Below is a velocity-time graph for a parachute jump.
Describe what happens to the
acceleration from a to c
- The gradient of the graph is decreasing.
- gradient of a v-t graph is the acceleration
- acceleration is decreasing
The anticlockwise moment acting the beam below is calculated by
M = F x perpendicular d
M = 15N x 0.2m = 3 Nm
Why was the distance 0.2 m used?
0.2 m is the perpendicular distance to the line of force.
OR
0.3 m is not perpendicular to the force
If the speed of a vehicle is doubled, what happens to the thinking distance.
As speed doubles, thinking distance doubles- you cover double the distance in the same reaction time.
What equipment is needed to study how the force on a spring affects its extension?
How would this be carried out accurately?
- read the length of the spring at eye level to avoid a parallax error
- allow spring to stop bouncing up and down before taking reading.
- repeat readings AND take an average by taking readings as the spring is loaded then again as it is unloaded- do not go beyond its elastic limit.
Below is a force-extension graph for a spring.
(a) In which portion of the graph does the spring obey Hooke’s Law?
(b) Where is the elastic limit (E) of the spring- where the spring would be permanently deformed?
The straight region of the graph shows that the force is proportional to the extention. Therfore, it obeys Hooke’s Law
If the spring is stretched beyond the elastic limit it would no longer go back to its original length.
Below is a velocity-time graph for a parachute jump.
What portion/s of the graph represents the greatest deceleration?
portion d
The velocity is decreasing at d and the gradient is very steep representing a very large deceleration.
How would you investigate how the force on a wire affects its extension?
- wire must be over 1 metre
- attach tape to wire with a pen line- read length at eye level
- add mass carefully to wire without pulling on it
A moment is the turning effect of a force. what factors affect the turning force?
The force exerted
The perpendicular distance between the line of action (force) and the pivot.
Moment = Force x perpendicular Distance
If the single spring needs 6 N to stretch 20 cm, how much force must hang on the parallel spring system to stretch it by the same amount (20 cm)?
There are two springs in parallel. Each spring needs 6N to stretch 20 cm. The overall force needed is 12 N to stretch the parallel system 20 cm
Below is a velocity-time graph for a parachute jump.
What portion/s of the graph represents the greatest deceleration?
portion d
The velocity is decreasing at d and the gradient is very steep representing a very large deceleration.
Below is a velocity-time graph for a parachute jump.
Describe what happens to the
acceleration from a to c
- The gradient of the graph is decreasing.
- gradient of a v-t graph is the acceleration
- acceleration is decreasing
List these people in order of stability.
Greatest to the least stable
Boy > teenager > woman > man
- The man has the highest centre of gravity*
- The boy has the lowest centre of gravity*
If a spring stretches by x centimeters with 1N hanging on it. How far will it stretch with 2N?
The spring will stretch 2x centimetres.
- Springs obey Hooke’s Law.*
- If force is doubled, extension doubles.*
- Force is proportional to extension*
What force is needed to balaance the beam?
M clockwise = 200 N x 40 cm = 800 Ncm
To be at equilibrium, sum of clockwise moments must equal the sum of hte anticlockwise moments
M anticlockwise = F x 100 cm = 800 Ncm
Force of 8 N needed!!
What force is needed to balance the beam?
M clockwise = 5 N x 0.5 m = 2.5 Nm
To be at equilibrium, sum of clockwise moments must equal the sum of hte anticlockwise moments
M anticlockwise = F x 0.25 = 2.5 Ncm
Force of 10 N needed!!
Below is a velocity-time graph for a parachute jump.
Explain the change in
acceleration from a to c
- at the start- only downward weight is acting on the person. Large resultant force downward. F=ma, large force –> more acceleration
- as speed increases at b, air resistance increases. Resultant force is smaller, F=ma, smaller force, less acceleration
- at c- upward air resistance = downward weight. Forces are balanced. Resultant force = zero, F=ma, no resultant force, no acceleration- constant speed and terminal velocity has been reached
Which exerted force (A, B or C) will create the largest moment (or turning effect) on the door?
B
It has the largest perpendicular distance to the pivot.
Moment = Force x perpendicular distance to the pivot
Both A and C have a zero perpendicular distance to the pivot
Does a rubber band obey Hooke’s Law?
How do you know?
The force extension graph for the rubber band is not a straight line through the origin.
Force is not proportional to extension
It does not obey Hooke’s Law
The graph below is the force-extension graph for a spring. Explain how you know that the spring obeys Hooke’s Law.
The force-extension graph is a straight line through the origin.
As the force doubles the extension doubles
Force is proportional to extension
How are velocity, displacement and time related?
velocity = displacement/ time
Is moment (turning effect) a vector or scalar quantity?
vector quantity
direction matters!!
A clockwise moment can cancel an anticlockwise moment.
